




 |
 |
 |
 |
 |
 |
| Click on images above to magnify. |
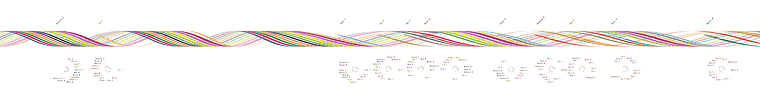
The results of a sample run are graphed. I left the controls on autopilot, so the evolution depicted in the above graph is solely due to the browser's own growth algorithms. I started with a list of keywords chosen from a New York Times article of August 08, 1999: Holbrooke, Balkans, peace, ethical, backwater. The maximum number of keywords was 12, so if a new keyword exceeded this limit, one from the existing list was dropped.
At the beginning of the run, coherence of the 'theme' of the set was maintained. New keyword members (Bosnia, Dayton, war, Sarajevo, Herzegovina, Serb, Mladic, Milosevic, Kosovo, nationalism, ...) were distinctly related to the initial set. Then the coherence started to fritter away. In came words with broader associations (government, CNN, 1996, 1999, issue, police, ...). As the run progressed, one could detect minor pockets of coherence, some vaguely related to the initial theme (Secretary, defense, troops, leader), but most further away (Tech, software, biocomputing, bioethics; Tan, Dun, Chinese, Heaven, music; Craig, Knoblock, series). Even later, associations became weaker. This behavior was typical of most runs left on autopilot. Of course, a little user input could counteract such entropy.
1. This graph can scale itself. That is, it has the ability to reveal more or less detail by loosening or tightening its helical coils. To reveal more activity, the graph 'relaxes.' Most maps or graphs must offer an additional frame at a different scale in order to offer more detail.
2. A remark on the problem of graphing: Graphing is the attempt to spatialize data in such a way that information is communicated. The success of a graph is evaluated by how well the information is communicated. Graphing must give dimension to data and numbers, which inherently have no spatial dimension (ignoring trivial cases where the data is spatial measurement, like 12 ft.) What makes graphing an interesting problem is that the process through which one gives concreteness to data usually introduces some distortion, and this distortion can impart extra, often unintended, information.
In the above graph, the problem faced is graphing the evolution of members of a set. The members of the set change with time, so obviously the dominant axis of the graph is time. Secondly, each member of the set has a weight, so that each item must be given another dimension. In the graph, this dimension is spatialized as radial arc length, or band width.
Spatializing the data poses a problem to the creator of the graph. When the data initially has no dimension, there is no problem with *where* things happen. However, in graphing the data, the placement of an item impacts its spatial relationship to the other items on the graph, also possibly affecting the information imparted by the graph.
In the case of the graphs above, where the relations of the members are changing with time, the graph must be able to convey these dynamic relations in a graphic way. As mentioned before, the weight of each keyword is represented by its band width. Furthermore, at any instant in time, the members must be distributed in space, so as not to be on top of each other. Thus each member has unique position in addition to extent.
Lastly, I arbitrarily imposed a continuity between the sequence of events in the graph, since this is how we're used to seeing events develop in time. In order to do this, judgments had to be made on how to depict transitions between events. As a result, an interesting side effect is that events seem to be *anticipated*. As keywords entered and exited the graph, existing keywords had to make room for these changes. Because of the desired continuity between changes, 'making room' involved transition over a time interval. Thusly existing members 'anticipated' newer members by making room for them. This is an example of how data can be given an extra reading by the way in which it is rendered spatially intelligible.
3. The problem of graphing can be seen as analogous to the problem of perception. As with graphing, the process of perception is making sense of data by means of 'imaging' the data. A tantalizing description of the process of perception is given by Alfred North Whitehead in Process and Reality: (Greatly simplified,) the initial datum is felt by the organism, the datum is supplemented with additional feelings and information, and integrated into a unified experience for the organism. In the supplementation and unification of data, data is made intelligible. Commonly, intelligibility involves spatialization of data.
As mentioned in Remark 2. /\, there is a certain amount of freedom in how the data is depicted. Judgments, partly aesthetic, were made to best describe the data. One may wonder, however, if a graph could have been made which would have been more homologous to the actual sequence of events. That is, could we make a graph which more accurately portrays the 'reality' of the events. Probably, but this does not detract from the comparison of the processes of perception and graphing. For, even if we imagine a certain aesthetic freedom is allowed even at the level of brute perception, that is, if the act of perception is 'colored' by decisions, even at levels way below consciousness, then such decisions would be akin to graphing decisions, and in a similar way they could have a bearing on the perceived reading of events. That is, such aesthetic freedoms could introduce an unknown amount of distortion.
Wittgenstein equated aesthetics and morals, and I see now what he may have meant. What we choose to see determines what we choose to believe, and certainly our morals color our perceptions.
4. An example of a distortion introduced in perception is motion blur. Suppose you watch a bird fly quickly past you. Your eye samples visual data at a certain rate so only certain points along the bird's trajectory are actually sensed. The process of perception fills in the rest with motion blur. It is a result of our impulse towards continuity in experience. Motion blur is so embedded in our experience that we impose it in our static depictions of motion, as for example in comic books. (For more on this phenomena, including its relation to film and computer games, see an essay, 30 vs 60 Frames Per Second >>, by Joshua Walrath.)